Slant Asymptote Lines + Special Case: Rational Functions and Long Division

0.458

IPFS

Open in the desktop app ADD TO PLAYLIST

Published on Nov 21, 2020

About :

In this video I go over Slant Asymptotes as well as the special case of finding Slant Asymptotes of Rational Functions through Polynomial Long Division. I actually briefly covered Slant asymptotes in my earlier video, so please ignore my statement in the video in which I said I should have covered this a long time ago, nonetheless I didn’t actually prove the special case in my earlier video. A slant asymptote is just that, an asymptote that is slanted, which is to say neither vertical nor horizontal. In other words a slanted asymptote is a linear function, and a function is said to have an slanted asymptote if it approaches the slanted line as x approaches positive or negative infinity. We can write this mathematically as the limit as x approaches infinity of the DIFFERENCE between the function and a linear function is equal to 0; i.e. the function is approaching the same value of the linear function.

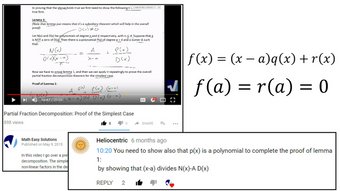
The special case for slant asymptotes occurs for Rational Functions in which the degree (or highest power) of the numerator is ONE MORE than the degree of the denominator. Recall that a rational function is a division of two polynomials on the domain that the denominator is not equal to zero. Thus when we use polynomial long division to divide out these two polynomials, I show that we end up with a quotient that is in fact a linear function, i.e. has a degree of 1. And combined with the fact that the remainder has a degree less than the divisor or denominator, as shown by Euclidean Division for Polynomials, then the difference between the function and the linear quotient is simply equal to the remainder divided by the denominator. Now the limit of the latter, as I show in the video, is equal to 0 because the higher power denominator approaches infinity “faster” than the remainder thus obtaining a 1 divided by infinity scenario, i.e. approaches 0. And this proves the special case and the quotient is the linear function which is the slant asymptote.

This is a very detailed video into slant asymptotes, especially the derivation of the special case, so make sure to watch this video!

Download the notes in my video: https://1drv.ms/b/s!As32ynv0LoaIh5AAP5jQxzqGnaj_ww

View video notes on the Hive blockchain: https://peakd.com/mathematics/@mes/slant-asymptote-lines-special-case-rational-functions-and-long-division