Conics in Polar Coordinates: Unified Theorem: Parabola Proof
1
About :
In this video I go over further into Conics Sections in Polar Coordinates and this time prove that the Unified Theorem for Conics does in fact apply for parabolas. The proof for parabolas is actually very simple because the Unified Theorem is derived very similarly to the conventional theorem for parabolas. The Unified Theorem states that the ratio of the distance from the conic to a fixed point F (called the focus) divided by the distance from the conic to a fixed line L (called the directrix), |PF|/|PL| = e (called the eccentricity) is equal to 1 for parabolas; thus e = 1. This means that |PF| and |PL| are “equidistant” or the same distances. This result is in fact the exact same as the conventional definition of a parabola which states that a parabola is the set of points that are equidistant from the focus and the directrix. Thus the ratio of the distance to the focus over the distance to the directrix must be 1 if they are equal. While this proof is very simple, the proofs for the ellipse (e is less than 1) and hyperbolas (e is less than 1) are more complicated so make sure to stay tuned for those proofs!
Also, as explained in this and prior videos, the main reason for using this more unified approach to define conics is that we can then combine the conics into a single formula using polar coordinates. This is a great video in understanding how different theorems can be identical depending on the perspective you view them as, so make sure to watch this video!
Download the notes in my video: https://1drv.ms/b/s!As32ynv0LoaIh5l1m5vRE5I2qmWDMw
View video notes on the Hive blockchain: https://peakd.com/mathematics/@mes/conics-in-polar-coordinates-unified-theorem-parabola-proof
Related Videos:
Conics in Polar Coordinates: Variations in Polar Equations Theorem: https://youtu.be/ud5f4C4pkpk
Conics in Polar Coordinates: Unified Theorem for Conic Sections: https://youtu.be/eUvzyxCfJCw
Conic Sections: Hyperbola: Definition and Formula: https://youtu.be/UBIHovXNV9U
Conic Sections: Ellipses: Definition and Derivation of Formula (Including Circles): https://youtu.be/9dETsJ2tz_M
Conic Sections: Parabolas: Definition and Formula: https://youtu.be/kCJjXuuIqbE
Polar Coordinates: Cartesian Connection: https://youtu.be/HcaTYrpmGaU
Polar Coordinates: Infinite Representations: https://youtu.be/QJYbnO7NzCk
Polar Coordinates: https://youtu.be/-KAdZL-N4ok .
SUBSCRIBE via EMAIL: https://mes.fm/subscribe
DONATE! ʕ •ᴥ•ʔ https://mes.fm/donate
Like, Subscribe, Favorite, and Comment Below!
Follow us on:
Official Website: https://MES.fm
Hive: https://peakd.com/@mes
Gab: https://gab.ai/matheasysolutions
Minds: https://minds.com/matheasysolutions
Twitter: https://twitter.com/MathEasySolns
Facebook: https://fb.com/MathEasySolutions
LinkedIn: https://mes.fm/linkedin
Pinterest: https://pinterest.com/MathEasySolns
Instagram: https://instagram.com/MathEasySolutions
Email me: contact@mes.fm
Try our Free Calculators: https://mes.fm/calculators
BMI Calculator: https://bmicalculator.mes.fm
Grade Calculator: https://gradecalculator.mes.fm
Mortgage Calculator: https://mortgagecalculator.mes.fm
Percentage Calculator: https://percentagecalculator.mes.fm
Try our Free Online Tools: https://mes.fm/tools
iPhone and Android Apps: https://mes.fm/mobile-apps
Tags :
Their limit for today is $0!






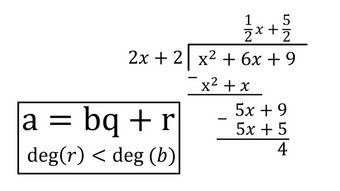



















Comments:
Reply:
To comment on this video please connect a HIVE account to your profile: Connect HIVE Account